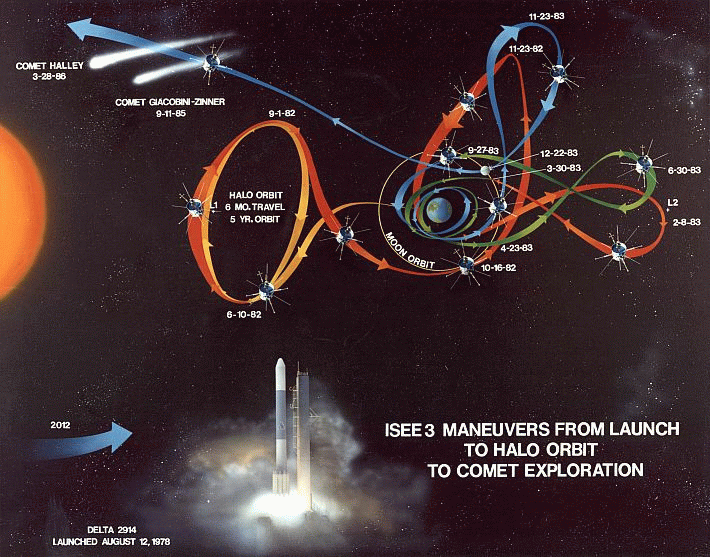
Last time I talked about a team trying to capture and reuse the ISEE3 satellite (here). The team has received lots of telemetry lately, determined the rotation speed of the satellite, and even had an amateur radio operator receive the satellite! While all of this is going on, they must rapidly plan out what orbit they wish to enter. The most discussed orbit is termed ESL1, the Earth-Sun system Lagrangian point #1. Lagrange points an interesting phenomena that I thought worth a short discussion.
When we think of orbits, traditionally we consult Kepler's laws. These "laws" are 3 simple rules that were written down between 1609 and 1619 by Johannes Kepler. I won't discuss them at length, because there are already many great sources to learn about Kepler's Laws and their application. The thing we want to draw from them is that an object orbiting closer to the Sun (say Venus), will have to travel faster to satisfy the laws of nature. In doing so it will orbit the Sun more times than the Earth will in the same amount of time. Venus will in fact orbit the sun 1.6 times during 1 orbit of the Earth!
Let's say we place a satellite far away from the Earth, between the Earth and sun. the satellite will orbit slightly faster than the Earth. Over a period of time it will be on the opposite side of the Sun and we won't be able to communicate. Eventually it will come around and lap the Earth! This isn't desirable, but we can use Lagrange points to solve this problem.
The simple laws of orbital mechanics that we have considered thus far are only valid for a simple problem with two objects (Earth and Sun or Earth and Satellite). We have we three bodies though, the Earth, the Sun, and the satellite! Three body problems are generally sticky to solve, but we have an advantage. The mass of a satellite is small compared to the mass of the Earth and the mass of the Sun (unless it's the Death Star). We can ignore the small mass of the satellite as solve what is known as the restricted three body problem. There are a few interesting points in space, the Lagrange points, at which the gravitational pull from the Sun and Earth are superimposed on each other to give the satellite the same orbital speed as the Earth!
The L1 point is where ISEE3 may end up, so let's look at it. The satellite will be above the Earth at an altitude of 1.5 million km (932,000 miles), towards the Sun. At this point, the two body mechanics say that the satellite will orbit the Sun faster than the Earth. Adding in the complications of the three body problem, we see that the gravitational tug of the Earth towards the Earth, away from the Sun is canceling out just enough of the Sun's pull to make the satellite orbit at the same angular speed as the Earth. How useful!
There are other Lagrangian points as well (L2-L5), but we won't discuss them here, other than to say that a similar explanation can be given for each. L4 and L5 are particularly interesting because they are inherently stable and hence lots of objects get caught there. There are objects in Earth-Sun L4/L5 and Earth-Moon L4/L5.
Generally satellites are placed in a small orbit around the L1 point for several reasons, including that it isn't inherently very stable. The ISEE3 team will have to execute a rather complex series of maneuvers to get to L1 again, using the pull of the moon and making a very close pass that comes within 10's of km of the surface of the moon. Time is of the essence, as the longer the wait the more they must change the speed of the craft (referred to as Delta V in the engineering jargon). The ship only has about 150m/s of Delta V left before it runs out of fuel. It'll take up to 1/3 of that to reposition the satellite, depending on how long the team must wait.
That's the quick and dirty view of Lagrangian points. I hope this was interesting and helps you understand space exploration, or your addiction to Kerbal Space Program a little more!